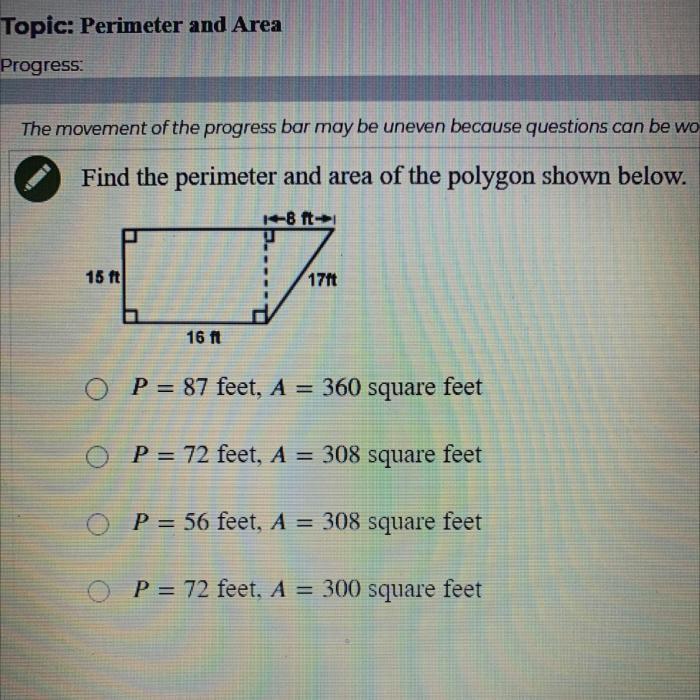
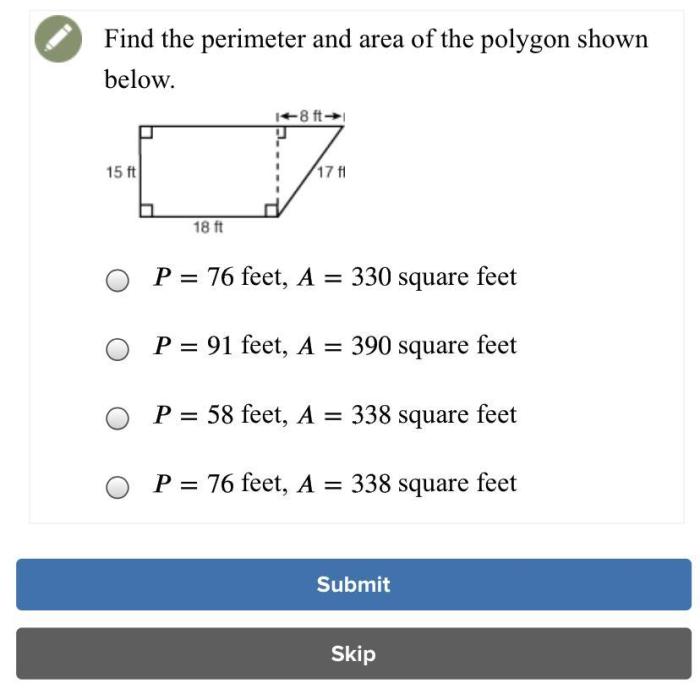
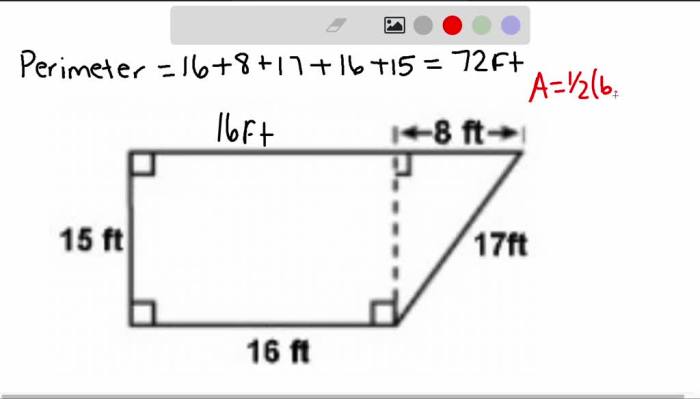
Find the perimeter and area of the polygon shown below. – Embarking on a journey to understand the perimeter and area of polygons, we delve into the fascinating world of geometry, where shapes come alive with mathematical precision. From defining these fundamental concepts to exploring their applications in various fields, this comprehensive guide will illuminate the intricacies of polygons, empowering you with a deeper understanding of their significance.

Polygons, ubiquitous in our surroundings, exhibit a rich diversity in their forms and properties. Understanding their perimeter and area equips us with the tools to quantify and analyze these captivating shapes, unlocking a wealth of knowledge about their characteristics and applications.

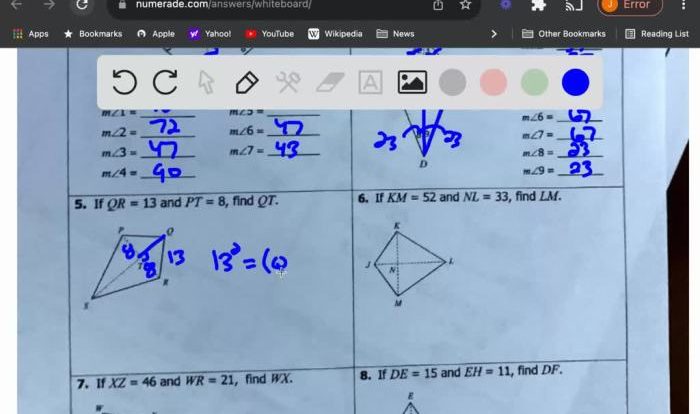
Perimeter: Find The Perimeter And Area Of The Polygon Shown Below.

The perimeter of a polygon is the total length of its sides.

To calculate the perimeter of a polygon, add up the lengths of all its sides. For example, the perimeter of a square with sides of length 5 cm is 5 cm + 5 cm + 5 cm + 5 cm = 20 cm.

Measuring the Perimeter, Find the perimeter and area of the polygon shown below.

• Use a ruler or measuring tape to measure the length of each side of the polygon.
• Add up the lengths of all the sides to find the perimeter.

Units of Measurement

The perimeter of a polygon is typically measured in units of length, such as centimeters, meters, or inches.

Area

The area of a polygon is the amount of space it encloses.

To calculate the area of a polygon, use the following formula:

Area = (1/2)

• base
• height

For example, the area of a rectangle with a base of 5 cm and a height of 3 cm is (1/2) – 5 cm – 3 cm = 7.5 cm ².

Measuring the Area

• Use a grid or graph paper to find the area of the polygon.
• Count the number of squares or grid units that are inside the polygon.
• Multiply the number of squares by the area of each square to find the area of the polygon.

Units of Measurement

The area of a polygon is typically measured in units of area, such as square centimeters, square meters, or square inches.

Polygon Types

Polygons can be classified into different types based on the number of sides they have.

Triangle

• 3 sides
• 3 vertices
• 3 angles

Quadrilateral

• 4 sides
• 4 vertices
• 4 angles

Pentagon

• 5 sides
• 5 vertices
• 5 angles

Hexagon

• 6 sides
• 6 vertices
• 6 angles

Polygon Properties

Polygons have certain properties that can be calculated based on the number of sides they have.

Number of Sides

The number of sides of a polygon is equal to the number of vertices it has.

Number of Vertices

The number of vertices of a polygon is equal to the number of sides it has.

Number of Diagonals

The number of diagonals in a polygon with nsides is given by the formula:

Number of diagonals = (n(n-3))/2

Applications of Polygons

Polygons are used in a variety of applications in architecture, engineering, and design.

Architecture

Polygons are used to create a variety of architectural structures, such as pyramids, domes, and arches.

Engineering

Polygons are used in the design of bridges, buildings, and other structures.

Design

Polygons are used in the creation of logos, patterns, and other design elements.

FAQ Resource

What is the formula for calculating the perimeter of a polygon?

The perimeter of a polygon is the sum of the lengths of all its sides.

How do I measure the area of an irregular polygon?

The area of an irregular polygon can be calculated using a grid or graph paper by dividing the polygon into smaller shapes with known areas.

What are the different types of polygons?

Polygons can be classified into various types based on the number of sides they have, such as triangles, quadrilaterals, pentagons, and hexagons.